After my comeback from the conference in Ghent (see here, here and here), I started a collaboration with Marco Ruggieri. Marco was instrumental in making me aware of that part of the community that does computations in QCD at finite temperature. The aim of these people is to get a full landscape of the ground state of hadronic matter, even when a magnetic field is applied and the vacuum state is expected to change. This is not just an intellectual exercise as recent observations of quark-gluon plasma are there to show and also some important experiments at LHC are now unfolding the complexity of this theory. So, we are saying about the forefront of modern research in the field of nuclear matter that can have significant impact in our understanding of the early universe.
As Marco pointed out in this blog (see here), due to the lack of knowledge of techniques to manage QCD at low-energies, we are not even able to give a definite answer to the question if different phases exist for hadronic matter and if a critical temperature, or a cross-over temperature, can be found from a theoretical standpoint. People use two different approaches to manage this question: lattice computations and phenomenological models like Nambu-Jona-Lasinio or sigma models. Lattice computations displayed a critical temperature at zero quark masses and zero chemical potential (see here) and a cross-over rather than a phase transition with non-zero quark masses. A critical temperature was found to be about 170 MeV. These studies are yet underway and improve year after year. From a theoretical point of view the situation is less clear even if a Nambu-Jona-Lasinio model can be used to work out a critical temperature. The model should be non-local.
With this scenario in view, it seems not thinkable a proof of existence of a critical point at zero quark masses and zero chemical potential. This is true unless we know how to manage the low-energy behavior of QCD. One should have solved the mass gap problem to say in a few words what is needed here. As my readers know, I am in a position to give a definite answer to such a question and, of course I did. On Friday I have uploaded a new paper of mine on arXiv (see here) and I have obtained an evidence for a critical point in QCD. This is the point in temperature where the chiral symmetry gets restored.
The idea for this paper come after I read a beautiful work by T. Hell, S. Roessner, M. Cristoforetti, W. Weise on the non-local Nambu-Jona-Lasinio model (see here). These authors completely work out the physics of this model. The point is that, as I have shown, this is the right one to describe low-energy physics in QCD. From a comparison with the form factors, mine obtained solving QCD with the mapping theorem and the one of Weise&al. guessed from a model of a liquid of instantons, the agreement is so good that my approach strongly supports the other view.
Finally, I was able to get the long sought equation for the critical temperature at zero quark masses and chemical potential. At this temperature the chiral symmetry appears to be restored. I find really interesting the fact that a similar equation was obtained by Norberto Scoccola and Daniel Gomez Dumm (see here). My equation for the critical temperature is substantially the same as theirs. Of course, the fundamental difference between my approach and all others relies on the fact that I am able to get the form factor solving QCD. In the preceding works this is just a guess, even if a very good one. Besides, so far, nobody was able to show that a Nambu-Jona-Lasinio model is the right low-energy limit of QCD. This result should be ascribed to me and Ken-Ichi Kondo (see here).
Having proved that a non-local Nambu-Jona-Lasinio model is the right low-energy limit, a prove of existence of a critical point is so obtained. This proof will be presented at the next conference in Paris on non-perturbative QCD (see here). Me and Marco will be there the next week.
Z. Fodor, & S. D. Katz (2004). Critical point of QCD at finite T and \mu, lattice results for physical
quark masses JHEP 0404 (2004) 050 arXiv: hep-lat/0402006v1
Marco Frasca (2011). Chiral symmetry in the low-energy limit of QCD at finite temperature arXiv arXiv: 1105.5274v2
T. Hell, S. Roessner, M. Cristoforetti, & W. Weise (2008). Dynamics and thermodynamics of a nonlocal Polyakov–Nambu–Jona-Lasinio
model with running coupling Phys.Rev.D79:014022,2009 arXiv: 0810.1099v2
D. Gomez Dumm, & N. N. Scoccola (2004). Characteristics of the chiral phase transition in nonlocal quark models Phys.Rev. C72 (2005) 014909 arXiv: hep-ph/0410262v2
The Gauge Connection 
Dear Marco,
I am sorry for being off topic, but I think it is worth asking.
For a safety margin, suppose that the SM is pretty much exact for thousands of TEVs. Now, the question:
Do the particles of the SM become massless above the electroweak scale?
Best,
Daniel.
Dear Daniel,
Yes, I think that electroweak symmetry is restored at higher energies. The question to be asked is if the Higgs model could be trusted or some other mechanism is at work. As you know, the scalar part of the Standard Model is suspected of triviality and so is essentially not meaningful from a physical standpoint becoming free of interactions.
Best,
Marco
Why becoming free of interactions? It seems that the quarks would become very similar to neutrinos. There would be some mass, since the symmetry should be allowed to be slightly broken due fluctuations of the energy. So, quarks would jump from generation to generation, like the neutrinos.
Higgs field, being trivial, becomes free of any interaction. The theory is meaningless. This is signaled by the presence of a Landau ghost in the running coupling of the theory. So, this part of the Standard Model is rather suspect from a theoretical point of view. It is possible that it is superseded by a supersymmetric Higgs model but, until LHC will not uncover it, we will not know.
By its side, when electroweak symmetry is restored, all the particles in the theory become massless. This was exactly the starting point of the theory.
Marco
Couldn’t the Landau ghost be an artifact of low order approximation?
Surely it is. But the triviality of this field is proved for dimensions greater or equal 5 and I proved this in four dimensions (if you cannot trust my conclusions there are lattice computations of course).
My conclusion is that, while integrating arbitrary terms of a perturbation series is an improper mathematical procedure to claim a global property, paradoxically that sign in a series term producing a claimed Landau ghost gives sense.
Marco
What about Top quark condensation? Well, why not condensate all quarks at the same time?
What are you aiming at? I do not see any sense at this. A top quark decays in a very short time and is unable to form bound states.
I am referring to this http://en.wikipedia.org/wiki/Top_quark_condensate
Daniel,
This is exactly Nambu-Jona-Lasinio model, nothing else. I hope we will find something more interesting at that energy scales even if, at the end of the day, all the possible mechanisms to form mass are already out.
Anyhow, interacting fermions to explain masses in the Standard Model is an old idea. You can find a good reference in this post .
Does the Higgs mechanism gives exactly massless particles or is it possible that some mass, a very small one remains?
In the Standard Model you have to be granted that the electroweak symmetry is an exact symmetry and this can only be obtained if all the particles in the model are exactly massless. Whatever mechanism you will use to break such a symmetry, you have to be granted that, once the symmetry is restored, then all the particles become exactly massless.
Marco
Can’t you relax that so that the masses are just null asymptotically?
Daniel,
Please, explain what is your aim. We will save time.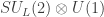
must be an exact symmetry. We know this from experiments that proved the model so successful. This also says to us that the symmetry is broken at lower energies due to the presence of the masses of the particles. This is the reason why people used mechanisms taken from phase transitions to assign such masses. But all these mechanisms should recover the exact symmetry increasing the energy scale and so particles must turn massless.
Marco
Neutrinos have 3 generations, but they jump from one to other, like they were just oscilating flavors. So, what if above the EW scale, quarks became identical, in this sence, to neutrinos. Small mass, an oscilating between generations.
Neutrinos would be a version of quarks that didn’t become too fat.
So, why should they oscillate? Some years ago some people conjectured such oscillations (e.g. neutron-antineutron) and some other baryon number violating processes. These were never seen. So, hope for the future.
I am thinking of something above the EW scale. Top, Strange and Down, for example, would have a mass similar to the neutrinos.
Dear Daniel,
Indeed, flavor mixing is a common observed effect. You can check a list of refs here.
Marco
[…] is a fundamental one and should be a rule. I have discussed this paper previously in my blog (see here and here) and I presented its content in a conference in Paris this year (see here). The […]